Step 1

Step 1 Description

The 2-D TGV test case has been already well documented in the scientific literature, e.g. . Therefore, only a brief description is given here. This configuration is used as verification step, since an analytic solution exists.

An incompressible flow with constant density and viscosity is simulated.

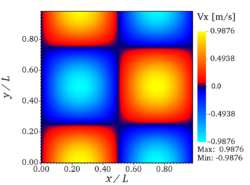
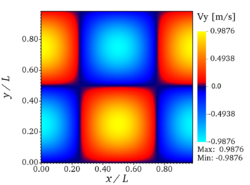
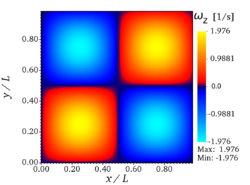
The fluid is contained in a square box of dimension $[0;L]^{2}$ with periodic boundary conditions in both directions. The value $L=2\pi L_{0}$ with $L_{0}=1\;\mathrm {m}$ has been arbitrarily chosen in the present benchmark for dimensional codes. The numerical value for the kinematic viscosity is chosen as $\nu =6.25\times 10^{-4}\;\mathrm {m^{2}/s}$ to get suitable conditions, as discussed below. The initial velocity components are prescribed as follows:

$u(x,y,0)=+u_{0}\times \sin(x/L_{0})\times \cos(y/L_{0})\,,$

$v(x,y,0)=-u_{0}\times \cos(x/L_{0})\times \sin(y/L_{0})\,.$

with $u_{0}=1\;\mathrm {m/s}$.

The flow is thus initially composed of four vortices, one in each quarter of the box. The vortex turnover time is defined as $\tau _{\mathrm {ref} }=L_{0}/u_{0}=1\;\mathrm {s}$. For non-dimensional codes, the relevant parameter for this simulation is the vortex Reynolds number, $Re=u_{0}L_{0}/\nu =1,600$ in the present setup. It should be noted that the theoretical solution is only stable for sufficiently small Reynolds numbers, explaining why the particular value $Re=1,600$ has been chosen here, as done in many previous studies using the TGV configuration.

A major interest of this test-case is that an analytical solution has been derived for such conditions:

$u(x,y,t)=u(x,y,0)\times \mathrm {e} ^{-2\nu t/L_{0}^{2}}=u(x,y,0)\times \mathrm {e} ^{-2\,(t/\tau _{\mathrm {ref} })/\mathrm {Re} }\,,$

$v(x,y,t)=v(x,y,0)\times \mathrm {e} ^{-2\nu t/L_{0}^{2}}=v(x,y,0)\times \mathrm {e} ^{-2\,(t/\tau _{\mathrm {ref} })/\mathrm {Re} }\,.$

All physical conditions are now completely defined and only numerical parameters remain to be set. Based on previously published studies, the recommended parameters for time and space discretization are $\Delta t=\tau _{\mathrm {ref} }/2000=5\times 10^{-4}\;\mathrm {s}$ and $N=64$ grid points in each direction. The flow must be simulated for a physical time of $t=10\;\tau _{\mathrm {ref} }=10\;\mathrm {s}$.

Aside suggestions

This step should clearly not be a big issue for any code. However, it is a good way to verify the orders of convergence (both in space and time), to check any stability issues, to measure the influence of the convergence criteria of iterative solvers (if any), etc...